
[Plot of a two-dimensional slice of the gravitational potential in and around a uniform spherical body. The inflection points of the cross-section are at the surface of the body.](By AllenMcC. - Own workThis mathematical image was created with Mathematica., CC BY-SA 3.0, Link)
First, we'll figure out what the potential is. Potential - the opportunity, the desire to make a move. This is the kind of description that comes to my mind when I hear the word potential. We say a person has the potential to "beautifully" compose poetry. So I understand it in such a way that in it is laid, or created, something out of this ability or opportunity, which under certain changes in surrounding conditions will manifest itself, in the form of writing ("creating") a poem. Hence, its potential is equal to one (conditionally!). I'm not interested in the process of folding words using all sorts of techniques. But I know for sure that he can do it. Another person does not have such a potential, which means that no matter how much he suffers, in the end he will not give birth to a poem. Its potential in this respect is zero (conditionally!).
Let us consider an example from classical physics. Our Earth, on the surface is a ball, measuring 1 meter cubic. Assume the ball consists of a substance that is naturally in the field of the Earth has a weight. The weight of the ball is 1 thousand conventional units. Weight is the strength with which our body presses on the support. What is its potential. And in that is the question, the potential for what. Because, as for the Earth, its potential is zero. But we perfectly understand that such a huge body and with such weight, can not have zero potential. And to properly build the logic of reflection, I came to the conclusion that there is no potential as such. BUT the potential difference has a place to be. Since the potential is a relative state from a reference point or, if desired, a reference point.
In our case, the ball lying on the Earth has the same potential as the Earth's surface. Therefore, the potential difference will be zero. But with respect to, for example, the Moon, its potential will not be already zero. Because our ball is at a distance from the moon. And this is what the conclusion suggests. The potential difference is, in fact, the distance between the interacting objects. The Earth and the sphere are attracted to each other - this is interaction. The distance between them is zero, and the potential difference is also zero.
Now take and transfer our ball to a distance of 1 conventional meter, notice I do not say "raise", but "transfer" what will be true when describing the potential and fix it in this position, let's put it under support. Now our ball is again motionless relative to the Earth, but its potential has changed. It became equal to 1 unit conditionally.
Now let's reflect. We know for sure that the force of action is equal to the force of opposition. According to Newton's third law. If the potential is a consequence of the interaction (again, not action, but mutual action), then the potential must have both bodies relative to each other. In this case, the earth's zero potential is not zero. A zero plays the role of the origin, for our convenience. In this case, the conclusion arises, if the potential difference is equal to unity and this difference is formed by two potentials, then the interaction center will be somewhere between these two objects. And the potentials of each object of the Earth and the cube will be greater than zero. And if the Earth's potential relative to the ball is not equal to 0, then the potential of the cube relative to the Earth will be greater than 1 (conventional unit). Of course, the possibilities of the Earth and the ball are not equal. The earth has much more potential, so our point (the center between the potentials) will be located practically on the surface of the Earth. BUT in reality, when we move the ball to a distance of 1 conventional meter, we move it a distance less because part of the way the Earth does in the opposite direction, as a result of our impact on the ball and the Earth (since the Earth is our support, in any case). It is not difficult to prove using the Law of Conservation. These movements remind us of pushing the bodies in opposite directions, when we are in the middle between them. Naturally, the body that moves more a very small distance, compared with the body is much smaller than it.
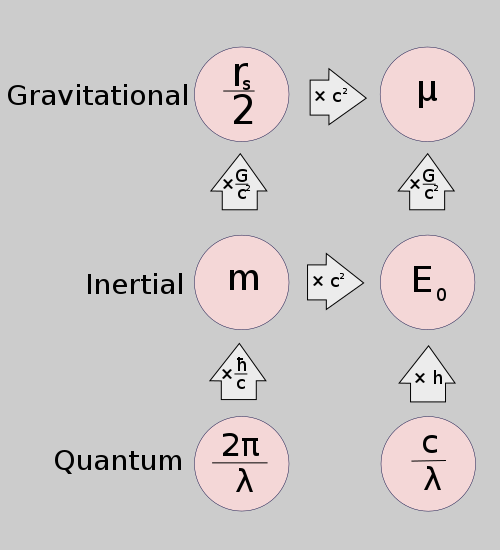
[The relation between properties of mass and their associated physical constants. Every massive object is believed to exhibit all five properties. However, due to extremely large or extremely small constants, it is generally impossible to verify more than two or three properties for any object. The Schwarzschild radius (rs) represents the ability of mass to cause curvature in space and time. The standard gravitational parameter (μ) represents the ability of a massive body to exert Newtonian gravitational forces on other bodies. Inertial mass (m) represents the Newtonian response of mass to forces. Rest energy (E0) represents the ability of mass to be converted into other forms of energy. The Compton wavelength (λ) represents the quantum response of mass to local geometry.](By Unitfreak - Own work by Unitfreak, Public Domain, Link)
In the beginning, we had three points.
The first point A. On the surface of the Earth
The second point B. On the surface of the sphere.
Third point D. Point along the middle of the line of interaction of two bodies.
All three points A, B and D coincide at the initial instant.
When the ball moves, the point D does not change its position on the line between two interacting bodies logically. So, according to our initial conditions, both the Earth and the Ball do not change during the entire experiment. The distance between the points A and B has changed as a result of the motion we have made. We will perform a mathematical calculation in an ideal system consisting of two bodies.
[Gravity field near the surface of the Earth – an object is shown accelerating toward the surface](By Lookang - Own work, CC BY-SA 3.0, Link)
The first body of the Earth has a mass of 6x10^21 conventional units.
The second body of the ball has a mass of 1 conventional unit.
The distance is 1 conventional meter.
F=Mm/r^2 (1)
The strength of the interaction is directly proportional to the product of the masses of the bodies and inversely proportional to the square of the distance.
In the potential form this formula will have the following form
φ=M/r (2)
As we agreed above, the potential difference is the distance between the interacting objects.
φ_1-φ_2=M/r_1 - m/r_2 =1 (3)
We solve equation 3. Even without making the calculation, it is easy to see that r_1 and r_2 will differ greatly, of the order of 10^10-10^11. Or in plain language, the Earth moves relative to our center at a distance comparable and slightly larger than the size of the atom. Whereas the ball is 1 meter away from the size of the same atom.
Let's conduct such a mental experiment. Can we move our Earth. We know the famous statement of Archimedes, "Give me support, and I can turn the Earth." As for me so much looks more like science advertising, probably even then scientists tried to attract the public to their craft. Nowadays, suppose that we made all people gather in one place. Now, on a command, people start to push off from the Earth and jump. Do it all at the same time. Assume and forces enough to jump up to 1 meter. But do they have enough power to move our Earth? Consider applying the formula 3. Only now the mass of the second body will be equal to the mass of all people, which is approximately 287 million tons for 2005, considering the mass of the adult population. To such a figure came British scientists estimated the total weight of all adults on Earth. (Source BMC Public Health)
The first body of the Earth has a mass of 2.1 x 10^13 conventional units.
The second body of the ball has a mass of 1 conventional unit.
The distance is 1 conventional meter.
The figures are different, but they will still be far from the units that the person operates in his daily life. Now the distance to which our Earth will move is commensurate with the size of a simple molecule, this is the order of 10x10^-7. It is natural to see such dimensions only in a microscope. It is not difficult to see if the body is repelled from the Earth and moves to a distance of 1 meter, whose weight is equal to the weight of the Earth, then in this case both bodies will shift to the same distance equal to 0.5 meters, from the initial point and in opposite directions.
Returning to Archimedes and his statement, it follows that in order to move the Earth, it is sufficient that the Earth itself will be the support, and that an impact of sufficient magnitude is necessary and the Earth will be shifted.

This post has been voted on by the steemstem curation team and voting trail.
There is more to SteemSTEM than just writing posts, check here for some more tips on being a community member. You can also join our discord here to get to know the rest of the community!
thanks @steemstem
Hi @leonid96!
Your post was upvoted by utopian.io in cooperation with steemstem - supporting knowledge, innovation and technological advancement on the Steem Blockchain.
Contribute to Open Source with utopian.io
Learn how to contribute on our website and join the new open source economy.
Want to chat? Join the Utopian Community on Discord https://discord.gg/h52nFrV
thanks for your support @utopian-io