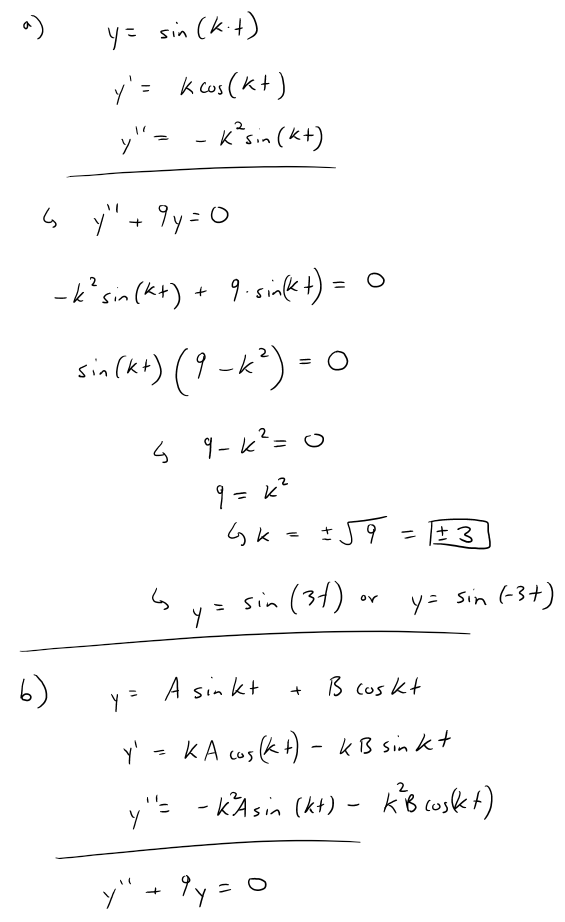In this video I go over an example on differential equations and this time show that the solutions to the second-order differential equation y" + 9y = 0 are in the form of a combination of sine and cosine functions. This differential equation can be re-arranged to y" = -9y which is the same form as that in my last video on the motion of a spring. And thus as expected the solutions should be involving trigonometric functions because they model the oscillations of a spring very well.
Watch on DTube:
Watch on YouTube: https://youtu.be/Twu30EJ93Wg
Watch on BitChute: https://www.bitchute.com/video/6WQIxUXca3Rs/ Watch on BitTube: https://bit.tube/play?hash=QmRLF5TuwPgfHxU6L7MmcToxShJr84evKD15NAs3sbShoD&channel=111547 Download PDF Notes: https://1drv.ms/b/s!As32ynv0LoaIhsE1Ms_uLy2M-31Bnw
View Video Notes Below!
Download These Notes: Link is in Video Description.
View These Notes as an Article: https://steemit.com/@mes Subscribe via Email: http://mes.fm/subscribe Donate! :) https://mes.fm/donateReuse of My Videos:
- Feel free to make use of / re-upload / monetize my videos as long as you provide a link to the original video.
Fight Back Against Censorship:
- Bookmark sites/channels/accounts and check periodically
- Remember to always archive website pages in case they get deleted/changed.
Join my private Discord Chat Room: https://mes.fm/chatroom
Check out my Reddit and Voat Math Forums:
Buy "Where Did The Towers Go?" by Dr. Judy Wood: https://mes.fm/judywoodbook Follow My #FreeEnergy Video Series: https://mes.fm/freeenergy-playlist Watch my #AntiGravity Video Series: https://mes.fm/antigravity-playlist Follow My #MESExperiments Video Series: https://mes.fm/experiments-playlist>
NOTE: If you don't have time to watch this whole video:
- Skip to the end for Summary and Conclusions (If Available)
- Play this video at a faster speed.
-- TOP SECRET LIFE HACK: Your brain gets used to faster speed. (#Try2xSpeed)
-- Try 4X+ Speed by Browser Extensions, HookTube.com, Modifying Source Code.
-- Browser Extension Recommendation: https://mes.fm/videospeed-extension- Download and Read Notes.
- Read notes on Steemit #GetOnSteem
- Watch the video in parts.
Modeling with Differential Equations: Motion of a Spring: Example 1
a) For what nonzero values of k does the function y = sin(kt) satisfy the differential equation y" + 9y = 0?
b) For those values of k, verify that every member of the family of the functions
y = Asin(kt) + Bcos(kt)
is also a solution.
Solution