Hi everyone. This post is about numbers and the number system in mathematics.
Sections
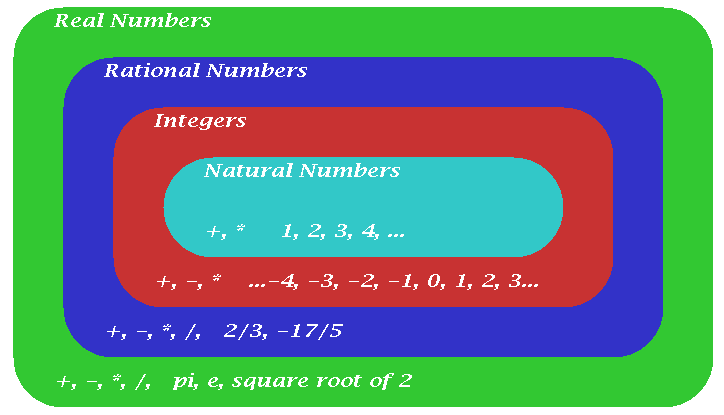
- Natural Numbers
- Non-Negative Integers
- Integers
- Rational Numbers (Fractions)
- Irrational Numbers
- Real Numbers
- Extension: Complex Numbers
Natural Numbers
The natural numbers refer to the positive whole numbers from 1 and going up. You could see them as counting numbers starting from 1. The set of all natural numbers is denoted by a funny looking N.
Non-Negative Integers
The non-negative integers is just an extension of the natural numbers. Instead of counting from 1, we start counting from 0 and then go up. The set of non-negative integers is {0, 1, 2, 3, 4, ... }.
Integers
When negative whole numbers are added to non-negative integers, we get the integers. The set of integers is denoted by a weird looking Z.
Rational Numbers (Fractions)
Many of us are familiar with the concepts of fractions. Fractions can also be referred to as rational numbers. A fraction/rational number contains an integer in the numerator (top) and a non-zero integer in the denominator (bottom). The set of rational numbers (fractions) is denoted by a strange looking Q.
This is the math version of saying that a and b are integers where the bottom cannot be zero (Dividing by zero is a math crime!).
Integers are considered rational numbers as the denominator/bottom of a fraction is simply 1 (b = 1).
Irrational Numbers
A number that is not rational (but still real) is irrational. Irrational numbers are not fractions and contain a never ending amount of decimal places. Examples include:
(Edit On Aug 12, 9:20PM EST: Fixed the golden ratio number typo. )
Real Numbers
All of the numbers (including the irrationals) from the previous sections belong to the real numbers. The real numbers is denoted by 
.
Extension: Complex Numbers
This particular concept may not be suitable for younger students. (You could just tell them that you cannot take the square root of a negative number as it is not a real number.)
Remember when a (high school) math teacher told you that a square root of a negative number is not real? It belongs to the set of complex numbers. The set of complex numbers is denoted by a weird looking C like this: 
.
A complex number contains a real number part and an imaginary number part. The form of a complex number is:
where
a is a real number
b is a real number
i is an imaginary number with 
.
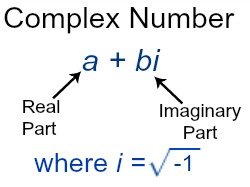
Complex numbers are used in fields such as physics, electrical engineering, fluid dynamics and certain math fields. I am not an expert on complex numbers so it is best to ask others who are familiar with complex numbers.
Here is another summary image but with the complex numbers.
I think the value of the golden ratio is wrong.
It is. Thank you for pointing it out. That extra 4 and 1 should not be there.
You could continue the diagram by adding quaternions and octonions as further supersets of complex numbers.
In addition, cardinals and ordinals are alternative ways of extending natural numbers, and p-adics are an alternative way of extending rationals into reals and beyond.
I do remember a little bit about cardinals, ordinals, countable sets, and uncountable sets from set theory.
I am not familiar with p-adics.
Even though I did learn a few things about complex numbers a while back such as Liouville's Theorem, line integrals and Laurent series, I have never heard of quaternions nor octonions.
Pretty good summary for all the families of number. Thank you the post.
Awesome, am curious to know irrational numbere like pi and e and many more.
Congratulations @dkmathstats! You have completed some achievement on Steemit and have been rewarded with new badge(s) :
Click on any badge to view your own Board of Honor on SteemitBoard.
For more information about SteemitBoard, click here
If you no longer want to receive notifications, reply to this comment with the word
STOP